[模板] 拓扑排序

Contents

1 定义
2 Kahn算法
3 例题 Luogu – 1038 神经网络

定义

拓扑排序是对一个 $DAG$ 图的线性排序算法，满足对于任何一条从 $u$ 到 $v$ 的有向边，都有 $u$ 在 $v$ 的前面

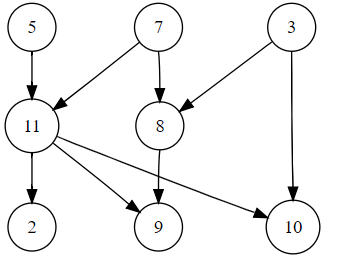
比如，下图的拓扑排序为 $3, 7, 8, 5, 11, 10, 2, 9$ （答案不唯一）

Kahn算法

将所有入度为 $0$ 的点插入队列 $S$ 中
当队列 $S$ 不为空的时候，去除队首 $x$，将 $x$ 插入数组 $ans$
删除所有从 $x$ 出发的有向边
检查有无新的入度为 $0$ 的点，插入队列 $S$ 中
回到第二步

最后 $ans$ 数组就是这个 $DAG$ 的拓扑排序

L ← Empty list that will contain the sorted elements
S ← Set of all nodes with no incoming edge
while S is non-empty do
    remove a node n from S
    add n to tail of L
    for each node m with an edge e from n to m do
        remove edge e from the graph
        if m has no other incoming edges then
            insert m into S
if graph has edges then
    return error   (graph has at least one cycle)
else 
    return L   (a topologically sorted order)

例题 Luogu – 1038 神经网络

题目描述

在兰兰的模型中，神经网络就是一张有向图，图中的节点称为神经元，而且两个神经元之间至多有一条边相连，下图是一个神经元的例子：

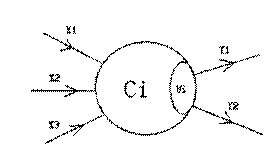
图中，$X_1 – X_3$ 是信息输入渠道，$Y_1 – Y_2$ 是信息输出渠道，$C_1$ 表示是阈值，可是为神经元的一个内在参数。

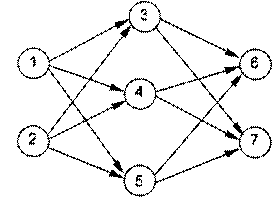
神经元按一定的顺序排列，构成整个神经网络。在兰兰的模型之中，神经网络中的神经元分为几层；称为输入层、输出层，和若干个中间层。每层神经元只向下一层的神经元输出信息，只从上一层神经元接受信息。下图是一个简单的三层神经网络的例子。

兰兰规定，$C_i$ 服从公式：(其中 $n$ 是网络中神经元的数目）

$$C_i = \sum_{j,i \in E} W_{ji}C_j – U_i $$

公式中的 $W_{ji}$ （可能为负值）表示连接 $j$ 号神经元和 $i$ 号神经元的边的权值，当 $C_i$ 大于 $0$ 时，该神经元处于兴奋状态，否则就处于平静状态。当神经元处于兴奋状态时，下一秒它会向其他神经元传送信号，信号的强度为 $C_i$

如此．在输入层神经元被激发之后，整个网络系统就在信息传输的推动下进行运作。现在，给定一个神经网络，及当前输入层神经元的状态（$C_i$），要求你的程序运算出最后网络输出层的状态。

输入格式

输入文件的第一行是两个整数 $n$ 和 $p$。接下来 $n$ 行，每行 $2$ 个整数，第 $i+1$ 行是神经元 $i$ 的最初状态和阈值，非输入层的神经元开始时的状态必然为 $0$。再接下来 $P$ 行，每行由 $2$ 个整数 $i, j$ 以及 $1$ 个整数 $W_{ij}$，表示连接神经元 $i, j$ 的边权为 $W_{ij}$

输出格式

输出文件包含若干行，每行有 $2$ 个整数，分别对应一个神经元的编号，及其最后的状态，$2$ 个整数间以空格分隔。仅输出最后状态大于 $0$ 的输出层神经元状态，并且按照编号由小到大顺序输出。

若输出层的神经元最后状态均为 $0$ ，则输出 “NULL”。

输入样例

输出样例

3 1
4 1
5 1

解题思路

我们统计每一个点的入度和出度，不难发现出度为 $0$ 的点一定是输出层。

将这幅图进行拓扑排序，对于每一个队首的点 $x$，对反向边全部做题目要求的公式操作，并对 $0$ 取最大值

最后输出结果即可

代码

#include <iostream>
#include <cstdio>
#include <cstring>
#include <cmath>
#include <algorithm>

using namespace std;
const int MAXN = 100 + 10;
const int MAXM = 20000 + 10;

int Head[MAXN], to[MAXM], Next[MAXM], w[MAXM], tot = 1;
bool vis[MAXN], flag[MAXN];
int in[MAXN], out[MAXN], ans[MAXN], u[MAXN], cnt;

inline int read(){
    int x = 0;
    int p = 1;

    char ch = getchar();

    while(ch < '0' || ch > '9'){
        if(ch == '-')
            p = 0;
        ch = getchar();
    }

    while('0' <= ch && ch <= '9'){
        x = x*10 + ch - '0';
        ch = getchar();
    }

    return p ? x : (-x);
}

inline void add(int a, int b, int c){
    to[tot] = b;
    w[tot] = c;
    Next[tot] = Head[a];
    Head[a] = tot++;
}

int que[MAXN], l, r;
int n, m;

inline void toop(){
    for(register int i=1; i<=n; i++){
        if(!in[i]){
            que[r++] = i;
            vis[i] = true;
        }
    }

    while(l != r){
        int x = que[l++];

        for(register int i=Head[x]; i; i=Next[i]){
            int v = to[i];

            if(vis[v]){
                ans[x] += ans[v] * w[i];
            }else{
                in[v]--;

                if(in[v] == 0){
                    que[r++] = v;
                }
            }
        }

        if(!flag[x]){
            ans[x] -= u[x];
        }

        ans[x] = max(ans[x], 0);
        vis[x] = true;
    }
}

int main(){
    n = read();
    m = read();

    for(register int i=1; i<=n; i++){
        ans[i] = read();
        u[i] = read();

        if(ans[i] != 0){
            flag[i] = true;
        }
    }

    for(register int i=1; i<=m; i++){
        int a = read();
        int b = read();
        int c = read();

        in[b]++;
        out[a]++;

        add(a, b, c);
        add(b, a, c);

    }

    toop();

    bool flag2 = false;

    for(register int i=1; i<=n; i++){
        if(ans[i] > 0 && out[i] == 0){
            printf("%d %d\n", i, ans[i]);
            flag2 = true;
        }
    }

    if(!flag2){
        puts("NULL");
    }
    return 0;
}

BLOG

定义